|
In this division problem, note that we have
annexed or added three zeros to help us divide. Each time we divide we get a remainder
of one. The decimal that we found (.333…)
is called a repeating
decimal because no matter how many
times we divide, our quotient will keep repeating. |
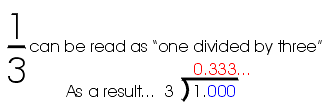
|